CRE's contribution to GAMM conference
The 94th Annual Meeting of the Society for Applied Mathematics and Mechanics (GAMM) was hosted by Otto von Guericke University Magdeburg in cooperation with the Max Planck Institute Magdeburg. The event took place from March 18th to March 22nd, 2024 in Magdeburg, Germany and Nataly Manque Roa and Xuan-Yi Zhang from Chair for Reliability Engineering represented the Chair and presented their research.
The Society for Applied Mathematics and Mechanics (German »Gesellschaft für Angewandte Mathematik und Mechanik« / GAMM) is an association that promotes scientific work across various disciplines such as Numerical Mathematics, Solid Mechanics, Aerodynamics and Perturbation Mechanics, Stochastics, Operations Research and Optimization and more. The annual conference serves as a significant platform for international scientific exchange.
Xuan-Yi Zhang’s Research:
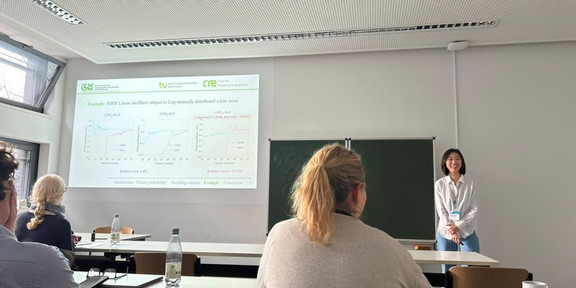
Advanced Directional Importance Sampling Method for Dynamic Reliability Analysis of Linear Structural System under Stochastic Non-Gaussian Loading
Abstract:
Reliability analysis of dynamic structural systems and its implications for structural design have garnered increasing attention. While Sample-based methods like Monte Carlo simulation prove inefficient due to their time-consuming computations, Directional Importance Sampling method showed promising results for linear structural systems, showcasing the ability to estimate small failure probabilities. However, DIS method was restricted to only linearity in Gaussian space and is not applicable to non-Gaussian problems.
This contribution introduces an advanced DIS method, referred to as ADIS, specifically designed for efficient dynamic reliability analysis of linear structural systems subjected to loading characterized as stochastic non-Gaussian white noise. To take the advantage of both linearity in physical space and simplicity of Gaussian space, directional importance sampling is conducted in Gaussian space and the failure probability is estimated with the aid of physical space. The information is exchanged between physical and Gaussian spaces with the aid of normal and inverse-normal transformation techniques. The whole procedure of the proposed ADIS method is straightforward with explicit estimator of the failure probability. The application of the proposed ADIS method is illustrated through a series of examples, illustrating its accuracy and efficiency for dynamic reliability analysis.
Nataly Manque Roa’s Research:
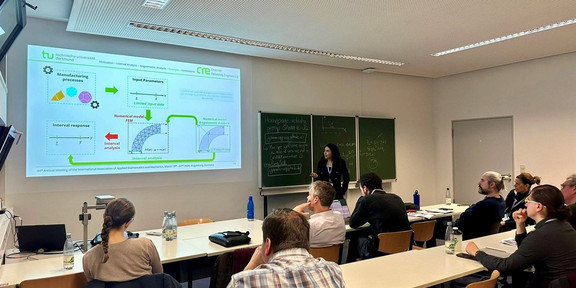
Application of Isogeometric Analysis for Interval Analysis
Abstract:
Geometrical uncertainty in manufacturing processes is often attributed to technology and operating conditions, resulting in discrepancies between numerical predictions and actual mechanical system behavior. The underlying cause is insufficient information during the design phase, which impedes robust numerical modeling due to uncertainty in system dimensions. Set-based methods such as intervals are useful for characterizing such uncertainties but can be computationally demanding when combined with traditional finite element methods. This study explores Isogeometric Analysis (IGA) for quantifying geometric uncertainties, which uses Non-Uniform Rational B-Splines (NURBS) to define the geometry and field solutions. The advantage of IGA is its ability to control the geometry through control points, thereby reducing the numerical cost of exploring different geometries. A gradient-based optimization algorithm is used to determine system response bounds, with sensitivities computed from the IGA model using a variational approach. A case study demonstrates the accuracy of the strategy in estimating uncertain stress triaxiality in a linear hook system with two uncertain geometric parameters.
The conference not only provided a chance to present the ongoing reseacrh but also to meet and exhange the ideas for future collaboration. We look forward to future collaboration.








